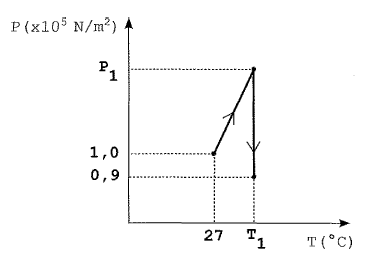
Um reservatório fechado contém certa quantidade de um gás ideal à pressão inicial P_o 1,00 times 10^5 N/m^2 . Num primeiro processo, esse gás é lentamente aquecido de T_o = 27, 0 °C até uma temperatura Ti. Num segundo processo, um pequeno orifício é aberto na parede do reservatório e, muito lentamente, deixa-se escapar 1/4 do conteúdo inicial do gás mantendo-se, porém, a temperatura constante (T_2 = T_1, ver gráfico). Sabendo que, ao final do segundo processo, a pressão do gás no interior do reservatório é P_2  = 0,900 times 10^5 N/m^2 , o valor de T_ 2, em °C, é

Let's analyze the problem step by step. In the first process, the ideal gas is slowly heated from an initial temperature of 27°C to a final temperature T₁. Since it's a quasi-static process, the temperature is uniform throughout the system. The ideal gas equation is given by PV = nRT, where n is the number of moles of the gas, R is the gas constant, and T is the temperature in Kelvin.

In the second process, a small orifice is opened in the reservoir, and 1/4 of the initial gas content is slowly released, maintaining the temperature constant at T₂ = T₁. Since the temperature is constant, the pressure will decrease as the gas is released.

From the ideal gas equation, we can write:

P₁V = n₁RT₁ and P₂V = n₂RT₂, where n₁ is the initial number of moles and n₂ is the final number of moles.

Since the volume is constant, we can divide both equations by V:

P₁ = n₁RT₁/V and P₂ = n₂RT₂/V

Now, we can use the given data:

P₁ = 1.00 × 10⁵ N/m², P₂ = 0.900 × 10⁵ N/m², and n₂ = 3/4n₁.

Substituting these values, we get:

1.00 × 10⁵ N/m² = n₁RT₁/V and 0.900 × 10⁵ N/m² = (3/4)n₁RT₂/V

Dividing both equations, we get:

T₂/T₁ = (4/3) × (P₂/P₁) = (4/3) × (0.900/1.00) = 1.20

Since T₁ = 27°C = 300 K, we have:

T₂ = 1.20T₁ = 1.20 × 300 K = 360 K = 87°C

Therefore, the correct answer is alternative E) 87.0°C.

Explanation: The key to this problem is to recognize that the temperature remains constant during the second process, allowing us to use the ideal gas equation to relate the initial and final pressures and temperatures.