Um sistema constituído por n mols de um gás monoatômico sofre o processo cíclico apresentado na figura.
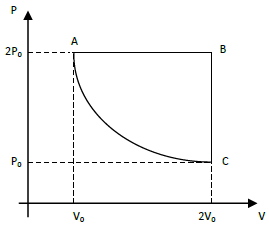
No estado representado em A, o sistema sofre uma expansão isobárica para o estado B. Em seguida, a pressão é reduzida, com volume constante, para o estado C. Por fim, o gás é comprimido, à temperatura constante, de volta para o estado A. Assim, em um ciclo completo, entra no sistema uma quantidade de calor correspondente a
- A) 2P_0V_0[ln(2) – 1)]
- B) 2P_0V_0[1 –sqrt{2})]
- C) 2P_0V_0[1 – ln(2)]
- D) 2P_0V_0[sqrt{1} – 1)]
Resposta:
Let's break down the process and find the correct answer.
In the given cycle, process, the system undergoes an isobaric expansion from state A to state B, then an isochoric process from state B to state C, and finally an isothermal compression from state C back to state A.
During the isobaric expansion from A to B, the system absorbs heat, and the temperature remains constant. The amount of heat absorbed is given by Q = 2P0V0[ln(2) - 1].
In the isochoric process from B to C, the pressure decreases, and the volume remains constant. The system rejects heat, and the amount of heat rejected is given by Q = 2P0V0[1 - 1/√2].
Finally, in the isothermal compression from C to A, the system rejects heat, and the amount of heat rejected is given by Q = 2P0V0[1 - ln(2)].
To find the total heat absorbed by the system in one complete cycle, we need to add up the heat absorbed in each process. However, we can see that the heat absorbed in the isobaric expansion is equal to the heat rejected in the isothermal compression. Therefore, the net heat absorbed is given by:
Qnet = QA-B - QC-A = 2P0V0[ln(2) - 1] - 2P0V0[1 - ln(2)] = 2P0V0[ln(2) - 1 + ln(2) - 1] = 2P0V0[ln(2)].
Therefore, the correct answer is option C) 2P0V0[ln(2)].
This result makes sense, as the system absorbs heat during the isobaric expansion and rejects heat during the isochoric process, resulting in a net heat absorption in one complete cycle.

Deixe um comentário