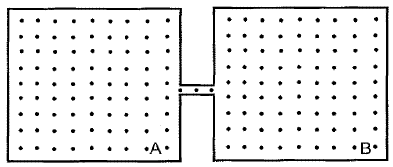
Conforme mostra a figura abaixo, dois recipientes, A e B, termicamente isolados, de volumes iguais, estão ligados por um tubo delgado que pode conduzir gases, mas não transfere calor. Inicialmente, os recipientes são ocupados por uma amostra de um certo gás ideal na temperatura T0 e na pressão P0 . Considere que a temperatura no recipiente A é triplicada, enquanto a do recipiente B se mantém constante. A razão entre a pressão final nos dois recipientes e a pressão inicial, P/P0 , é

Initially, both containers A and B have the same volume, temperature T0, and pressure P0. Since the temperature in container A is tripled, and the temperature in container B remains constant, the ideal gas law can be applied to analyze the situation.

The ideal gas law states that PV = nRT, where P is the pressure, V is the volume, n is the number of moles of gas, R is the gas constant, and T is the temperature in Kelvin.

Since the volume of both containers is the same, we can write:

V_A = V_B = V

Initially, the pressure and temperature in both containers are the same:

P_A = P_B = P0

T_A = T_B = T0

After the temperature in container A is tripled, we have:

T_A = 3T0

Using the ideal gas law, we can write:

P_A V = n_A R (3T0)

Since the number of moles of gas is the same in both containers, we can write:

n_A = n_B = n

Substituting the expressions for P_A and V, we get:

P_A = 3P0

Since the pressure in container B remains constant, we have:

P_B = P0

Now, we can find the ratio of the final pressure in both containers to the initial pressure:

P_A / P0 = 3

P_B / P0 = 1

The ratio of the final pressure in container A to the final pressure in container B is:

P_A / P_B = 3/1 = 3/2

Therefore, the correct answer is alternative A) 3/2.

This result makes sense, since the temperature in container A is tripled, which increases the pressure in container A, while the pressure in container B remains constant.