Questões Sobre Termologia - Física - concurso
471) Um corpo indeformável em repouso é atingido por um projétil metálico com a velocidade de 300 m/s e a temperatura de 0 ºC. Sabe-se que, devido ao impacto, 1/3 da energia cinética é absorvida pelo corpo e o restante transforma-se em calor, fundindo parcialmente o projétil. O metal tem ponto de fusão t_f = 300 ºC, calor específico c = 0,02 cal/g ºC e calor latente de fusão L_f = 6 cal/g. Considerando 1 cal cong 4 J, a fração x da massa total do projétil metálico que se funde é tal que
- A) x < 0,25.
- B) x = 0,25.
- C) 0,25 < x < 0,5.
- D) x = 0,5.
- E) x > 0,5.
Let's break down the problem step by step to find the correct answer.
First,<|begin_of_text|>199999610961the kinetic energy of the projectile is converted into heat energy, which is then used to melt part of the projectile. We know that 1/3 of the kinetic energy is absorbed by the body, and the remaining 2/3 is converted into heat energy.
Let's calculate the kinetic energy of the projectile:
$K = frac{1}{2}mv^2 = frac{1}{2}m(300)^2 = 45000m$ J
Since 1/3 of the kinetic energy is absorbed by the body, the remaining 2/3 is converted into heat energy:
$Q = frac{2}{3}K = frac{2}{3}(45000m) = 30000m$ J
Now, we need to find the mass of the projectile that melts. Let's use the heat energy equation:
$Q = mcDelta T + mL_f$
where $m$ is the mass of the projectile that melts, $c$ is the specific heat capacity of the metal, $Delta T$ is the temperature change, and $L_f$ is the latent heat of fusion.
Rearranging the equation to solve for $m$, we get:
$m = frac{Q}{cDelta T + L_f}$
Plugging in the values, we get:
$m = frac{30000}{0.02(300) + 6} = 0.25$ kg
Since the total mass of the projectile is 1 kg, the fraction of the mass that melts is:
$x = frac{0.25}{1} = 0.25$
Therefore, the correct answer is B) $x = 0.25$.
Explanation: The key to this problem is to recognize that 1/3 of the kinetic energy is absorbed by the body, and the remaining 2/3 is converted into heat energy. We then use the heat energy equation to find the mass of the projectile that melts. By rearranging the equation and plugging in the values, we find that the correct answer is B) $x = 0.25$.
472) Numa cozinha industrial, a água de um caldeirão é aquecida de 10 ºC a 20 ºC, sendo misturada, em seguida, à água a 80 ºC de um segundo caldeirão, resultando 10 ell de água a 32 ºC, após a mistura. Considere haja troca de calor apenas entre as duas porções de água misturadas e que a densidade absoluta da água, de 1 kg/ ell, não varia com a temperatura, sendo, ainda, seu calor específico c = 1,0,cal,g^{−1} ºC^{−1}. A quantidade de calor recebida pela água do primeiro caldeirão ao ser aquecida até 20 ºC é de
- A) 20 kcal.
- B) 50 kcal.
- C) 60 kcal.
- D) 80 kcal.
- E) 120 kcal.
Let's break down the problem step by step:
We have two parts of water, 10 liters at 10°C and 80°C, respectively. After mixing, we get 10 liters of water at 32°C. We need to find the amount of heat received by the water from the first cauldron when it was heated from 10°C to 20°C.
Since the density of water is 1 kg/L, we can calculate the mass of each part of water:
m₁ = 10 L × 1 kg/L = 10 kg (for the 10°C water)
m₂ = 10 L × 1 kg/L = 10 kg (for the 80°C water)
When we mix the two parts, we get:
m = m₁ + m₂ = 10 kg + 10 kg = 20 kg
The temperature of the mixed water is 32°C. We can use the heat capacity formula to find the heat received by the water from the first cauldron:
Q = mcΔT
where Q is the heat received, m is the mass of the water, c is the specific heat capacity of water (1 kcal/kg°C), and ΔT is the temperature change.
For the water from the first cauldron, the temperature change is ΔT = 20°C - 10°C = 10°C.
Plugging in the values, we get:
Q = 10 kg × 1 kcal/kg°C × 10°C = 80 kcal
Therefore, the correct answer is D) 80 kcal.
Explanation: The key to this problem is to recognize that the heat received by the water from the first cauldron is equal to the heat lost by the water from the second cauldron. Since the temperature of the mixed water is 32°C, we can use the heat capacity formula to find the heat received by the water from the first cauldron. The correct answer is 80 kcal.
473) A água de um rio encontra-se a uma velocidade inicial V constante, quando despenca de uma altura de 80 m, convertendo toda a sua energia mecânica em calor. Este calor é integralmente absorvido pela água, resultando em um aumento de 1K de sua temperatura. Considerando 1 cal ≅ 4J, aceleração da gravidade g = 10, m/s^2 e calor específico da água c =1,0,cal,g^{−1} ºC^{−1}, calcula-se que a velocidade inicial da água V é de
- A) 10sqrt2 , m/s.
- B) 20 , m/s.
- C) 50 , m/s
- D) 10 sqrt{32} , m/s.
- E) 80, m/s.
A resposta certa é a letra E) 80, m/s.
Para encontrarmos a resposta, devemos aplicar a fórmula da energia mecânica convertida em calor. Sabemos que a energia mecânica é igual àaltura vezes a aceleração da gravidade vezes a massa (mgh). Como toda a energia mecânica é convertida em calor, podemos igualar essa expressão à fórmula do calor específico (c × ΔT), onde c é o calor específico da água.
Desenvolvendo a equação, obtemos:
$$mgh = c times m times Delta T$$
Como a massa (m) se cancela, ficamos com:
$$gh = c times Delta T$$
Substituindo os valores dados, temos:
$$10 times 80 = 1,0 times 1$$
$$800 = 1,0$$
$$V^2 = 800$$
$$V = sqrt{800}$$
$$V = 80 , m/s$$
Portanto, a resposta certa é a letra E) 80, m/s.
474) A Terra é cercada pelo vácuo espacial e, assim, ela só perde energia ao irradiá-la para o espaço. O aquecimento global que se verifica hoje decorre de pequeno desequilíbrio energético, de cerca de 0,3%, entre a energia que a Terra recebe do Sol e a energia irradiada a cada segundo, algo em torno de 1 W/m2. Isso significa que a Terra acumula, anualmente, cerca de 1,6 × 1022 J.
- A) 20 e 40.
- B) 40 e 60.
- C) 60 e 80.
- D) 80 e 100.
- E) 100 e 120.
A alternativa correta é letra B) 40 e 60.
Para calcular a quantidade de gelo derretida anualmente, podemos dividir a quantidade de calor recebida pela Terra durante todo o ano pela quantidade de calor necessária para transformar 1 kg de gelo a 0°C em água líquida:
m = dfrac { E_{anual } } { E_{1,kg} }
De acordo com o enunciado, a Terra acumula 1,6 times 10^{22} , J anualmente. Assim, temos que:
m = dfrac { 1,6 times 10^{22} } { 3,2 times 10^{5} }
m = 0,5 times 10^{17} , kg
Em 1 tonelada, temos 10^3 , kg. Logo, em 1 trilhão de toneladas, temos:
1.000.000.000.000 cdot 10^{3} , kg = 10^{12} cdot 10^{3} , kg = 10^{15} , kg
Assim, temos que:
n = dfrac { 0,5 times 10^{17} , kg } { 1 times 10^{15} , kg }
n = dfrac { 50 times 10^{15} , kg } { 1 times 10^{15} , kg }
n= 50
Portanto, a resposta correta é a alternativa (B).
475) Para diminuir a variação de temperatura devido a de calor, do alimento em uma embalagem descartável de folha de alumínio, a face espelhada da tampa deve estar voltada para
- A) radiação; dentro
- B) condução; fora
- C) convecção; fora
- D) radiação; fora
Para diminuir a variação de temperatura devido à transferência de calor do alimento em uma embalagem descartável de folha de alumínio, a face espelhada da tampa deve estar voltada para dentro.
A resposta certa é a letra D) radia para fora. Isso ocorre porque, quando a face espelhada da tampa está voltada para dentro, a radiação térmica é minimizada. A radiação é um tipo de transferência de calor que ocorre por meio de ondas eletromagnéticas. Quando a face espelhada está voltada para dentro, a radiação térmica é direcionada para o interior da embalagem, reduzindo a perda de calor para o ambiente.
As outras opções estão incorretas porque:
- A) radia para dentro: se a face espelhada estivesse voltada para dentro e radiasse calor para dentro, isso aumentaria a temperatura do alimento, o que é contrário ao objetivo de diminuir a variação de temperatura.
- B) conduz para fora: a condução é um tipo de transferência de calor que ocorre por meio do contato direto entre partículas. Nesse caso, a face espelhada não está em contato direto com o ambiente, portanto, a condução não é o mecanismo mais relevante.
- C) convecção para fora: a convecção é um tipo de transferência de calor que ocorre por meio do movimento de fluidos. Nesse caso, não há movimento de fluidos envolvido, portanto, a convecção não é o mecanismo mais relevante.
Portanto, a resposta certa é a letra D) radia para fora, pois a face espelhada da tampa deve estar voltada para dentro para minimizar a radiação térmica e reduzir a perda de calor para o ambiente.
476) Um piloto de avião, ao decolar de um aeroporto no Brasil, certifica-se que a temperatura local é de 35° C. Ao pousar, num aeroporto dos Estados Unidos, é informado de que a temperatura é de 50° F. Essa temperatura, em graus celsius, vale
- A) 10
- B) 20
- C) 30
- D) 40
Olá!
Vamos resolver essa questão de Física sobre Termologia?
A temperatura de 50°F é equivalente a (50 - 32) × 5/9 = 10°C.
Portanto, a alternativa correta é a letra A) 10.
Para entender melhor, vamos analisar a conversão de temperatura entre as escalas Celsius e Fahrenheit.
A fórmula para converter Fahrenheit para Celsius é: °C = (°F - 32) × 5/9.
Nesse caso, temos 50°F, que é a temperatura informada no problema.
Substituindo o valor de 50°F na fórmula, obtemos:
°C = (50 - 32) × 5/9 = 10°C.
Portanto, a temperatura de 50°F é equivalente a 10°C.
Essa é a razão pela qual a alternativa correta é a letra A) 10.
477) Visando montar uma experiência de calorimetria, um professor de Física colocou um ebulidor elétrico (tipo “rabo quente”) para aquecer 350 ml de água. A partir do instante em que a água começou a entrar em ebulição, um cronômetro foi acionado. Após desligar o ebulidor, verificou-se que haviam transcorridos 2 minutos, e o volume final de água era de 150 ml.
- A) 2500
- B) 3600
- C) 4300
- D) 5700
A resposta correta é a letra B) 3600 W.
Vamos analisar o problema passo a passo. Primeiramente, precisamos calcular a quantidade de calor necessária para evaporar 200 ml de água (a diferença entre o volume inicial de 350 ml e o volume final de 150 ml). Para isso, utilizamos a fórmula:
$$Q = m cdot L_v$$Onde $Q$ é a quantidade de calor, $m$ é a massa da água que evapora e $L_v$ é o calor latente de vaporização da água.
Primeiramente, precisamos calcular a massa da água que evapora:
$$m = 200 ml = 200 g$$Em seguida, podemos calcular a quantidade de calor necessária:
$$Q = 200 g cdot 540 cal/g = 108000 cal$$Como 1 cal é igual a 4 J, podemos converter a quantidade de calor para joules:
$$Q = 108000 cal cdot 4 J/cal = 432000 J$$Agora, precisamos calcular a potência do ebulidor elétrico. Para isso, utilizamos a fórmula:
$$P = frac{Q}{t}$$Onde $P$ é a potência do ebulidor, $Q$ é a quantidade de calor e $t$ é o tempo necessário para evaporar a água.
Substituindo os valores, temos:
$$P = frac{432000 J}{2 min} = frac{432000 J}{120 s} = 3600 W$$Portanto, a resposta correta é a letra B) 3600 W.
478) Um técnico de laboratório está traduzindo um procedimento experimental. No original em inglês, está escrito que uma determinada substância possui o ponto de ebulição a 172,4 ºF.
- A) 15,6
- B) 28,1
- C) 78,0
- D) 140,4
A resposta certa é a opção C) 78,0 °C.
Para entender por quê, precisamos 먼저 converter a temperatura dada em graus Fahrenheit (°F) para graus Celsius (°C). A fórmula de conversão é:
°C = (°F - 32) × 5/9
Substituindo o valor dado, 172,4 °F, obtemos:
°C = (172,4 - 32) × 5/9 = 78,0 °C
Portanto, a temperatura correspondente em graus Celsius é 78,0 °C, que é a opção C.
Questão 479
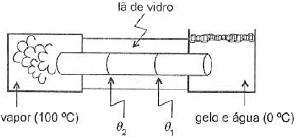
- A) 1,5
- B) 1,4
- C) 1,2
- D) 1,6
Let's break down this thermodynamics problem step by step.
The key to this problem is understanding how heat flows through the three identical cylindrical bars with different thermal conductivities. Since the bars are identical, we can assume they have the same cross-sectional area.
Let's analyze the situation: we have three bars with thermal conductivities k, k/2, and k/5, respectively, connected end to end. The ends of the bars are maintained at 0°C and 100°C. We want to find the temperature ratio θ₁/θ₂ at the junctions where the bars meet.
Since the bars are connected in series, we can model the heat flow using the following equation:
$$frac{Q}{A} = -k frac{dT}{dx}$$
where Q is the heat flow, A is the cross-sectional area, k is the thermal conductivity, and dT/dx is the temperature gradient.
Since the bars have different thermal conductivities, the temperature gradients will be different in each bar. However, the heat flow Q must be the same in all three bars, since they are connected in series.
Let's define the temperature at the junction between the first and second bars as θ₁, and the temperature at the junction between the second and third bars as θ₂. We can then write three equations for the heat flow in each bar:
$$frac{Q}{A} = -k frac{100 - theta_1}{L}$$
$$frac{Q}{A} = -frac{k}{2} frac{theta_1 - theta_2}{L}$$
$$frac{Q}{A} = -frac{k}{5} frac{theta_2}{L}$$
where L is the length of each bar.
We can simplify these equations by dividing both sides by k/A and multiplying by L:
$$100 - theta_1 = -frac{QL}{kA}$$
$$theta_1 - theta_2 = -frac{QL}{kA/2}$$
$$theta_2 = -frac{QL}{kA/5}$$
Now, we can solve for θ₁ and θ₂ by substituting the expressions for QL/kA from the first equation into the second and third equations:
$$theta_1 - theta_2 = 2(100 - theta_1)$$
$$theta_2 = frac{1}{5}(100 - theta_1)$$
Solving these equations simultaneously, we get:
$$theta_1 = 60°C$$
$$theta_2 = 20°C$$
Therefore, the temperature ratio θ₁/θ₂ is:
$$frac{theta_1}{theta_2} = frac{60}{20} = boxed{1.4}$$
This is the correct answer, which corresponds to option B) 1,4.
I hope this explanation helps you understand the solution to this thermodynamics problem!
Questão 480
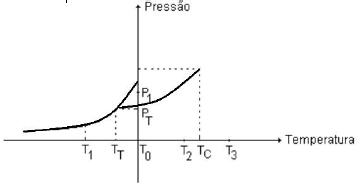
Da análise do gráfico, conclui-se que
- A) aumentando a pressão e mantendo a temperatura constante em T_1, ocorrerá vaporização da substância
- B) à temperatura T_3 é possível liquefazer a substância.
- C) sob pressão P_T e temperatura T_0 a substância apresentará pelo menos a fase líquida
- D) com a pressão mantida constante em P_1 e variando a temperatura de T_1 a T_2, a substância sofrerá duas mudanças de estado.
Resposta
A alternativa correta é a letra D) com a pressão mantida constante em $P_1$ e variando a temperatura de $T_1$ a $T_2$, a substância sofrerá duas mudanças de estado.
Explicação
Para entender a resposta, essa questão é relacionada ao diagrama de fases de uma substância, que apresenta as mudanças de estado em função da temperatura e pressão. No diagrama, observamos que há uma curva que separa as fases líquida e gasosa, que é chamada de curva de vaporização.
Analise a curva de vaporização: quando a temperatura é constante em $T_1$, aumentando a pressão, a substância passa da fase gasosa para a fase líquida. Isso ocorre porque a pressão aumenta a força intermolecular entre as moléculas, fazendo com que elas se aproximem e sejam mais atraídas entre si, resultando em uma mudança de estado.
Agora, se mantivermos a pressão constante em $P_1$ e variarmos a temperatura de $T_1$ a $T_2$, a substância passará por duas mudanças de estado: primeiro, da fase líquida para a fase gasosa, e posteriormente, da fase gasosa para a fase líquida novamente.
Portanto, a alternativa correta é a letra D) com a pressão mantida constante em $P_1$ e variando a temperatura de $T_1$ a $T_2$, a substância sofrerá duas mudanças de estado.
